Solving a Birkhoff Interpolation Problem for (0, 1, 5) Data

Dr. Rama Nand Mishra; Dr. Akhilesh Kumar Mishra; Dr. Kulbhushan Singh; Dr. Arunesh Kumar Mishra

doi:10.54060/a2zjournals.jase.30

Authors

Dr. Rama Nand Mishra Faculty of Mathematical and Statistical Sciences, Sri Ramswaroop Memorial University, Lucknow-Deva Road, Bara-banki, Uttar Pradesh, India
Dr. Akhilesh Kumar Mishra Department of Applied Sciences, Brahma Valley College of Engineering & Research Institute, Anjaneri, Nashik, Maha-rastra, India
Dr. Kulbhushan Singh Faculty of Mathematical and Statistical Sciences, Sri Ramswaroop Memorial University, Lucknow-Deva Road, Barabanki, Uttar Pradesh, India
Dr. Arunesh Kumar Mishra Faculty of Mathematical and Statistical Sciences, Sri Ramswaroop Memorial University, Lucknow-Deva Road, Barabanki, Uttar Pradesh, India

DOI:

https://doi.org/10.54060/a2zjournals.jase.30

Keywords:

Spline function, Acidic, Birkhoff interpolation, AMS Classification: 65L10

Abstract

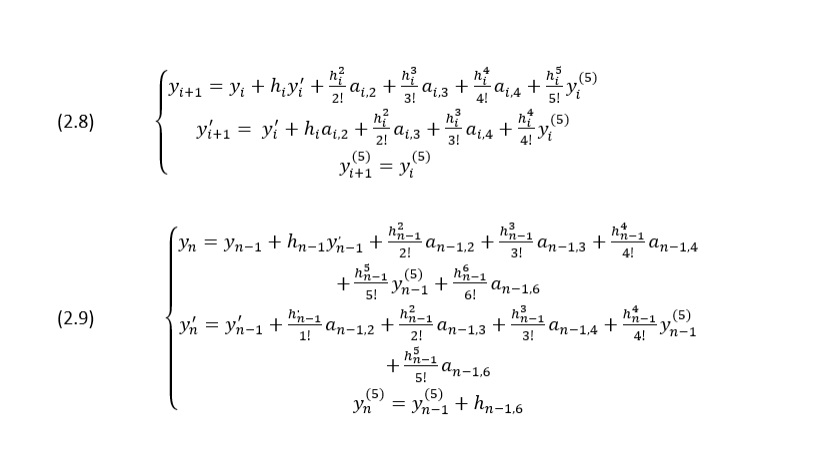
The following research work deals with a special type of Birkhoff’s interpolation problem in which we have 3 sets of data prescribed in the unit interval I = [0, 1]. Data values are the function value, first derivatives and fifth derivatives prescribed at nodes of the unit interval. We obtained a unique spline interpolating the given data along with the convergence problem.

Downloads

Download data is not yet available.

References

F. R. Loscalzo, T. D. Talbot, “Spline and approximation for solutions of ordinary differential equations,” SIAM J. Numer. Anal., vol. 4, pp. 433-445, 1967.

G. H. Micula, “Approximate solution of the differential equation y'' (x) = f(x,y) with spline functions,” Math. of comput, vol. 27 pp. 807-816, 1973.

T. Fawzy, “Spline functions and the Cauchy's problem II,” Acta Math. Hung, vol. 29, no. 3-4 pp. 259-271, 1977.

J. Gyorvari, “Lakunare spline funktionun das Cauchy problem,” Acta Math Hung, vol. 44, no. 3–4, pp. 327–335, 1984.

S. Sallam, M. A. Hussain, “deficient spline for approximation to second order differential equations,” Appl. Math Model-ing, vol. 8, pp. 408-412, 1984.

K. B. Singh, A. Pandey, and Q. Ahmad, “Solution of a Birkhoff Interpolation Problem by a Special Spline Func-tion,” International J. of Comp. App, vol. 48, pp. 22–27, 2012.

A. K. Pandey, Q. S. Ahmad, and K. Singh, “Lacunary Interpolation (0,2;3) Problem and Some Comparison from Quartic Splines,” American J. of App. Math. and Statistics, vol. 1, no. 6, pp. 117–120, 2013.