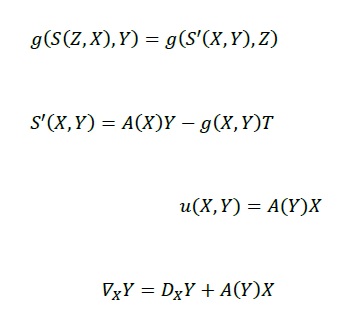Study of Some Properties on Almost Para Contact Metric Manifolds with Certain Connection
DOI:
https://doi.org/10.54060/JASE/001.01.006Keywords:
Semi-symmetric nonmetric connection, almost para contact metric manifold, Nijenhuis tensorAbstract
Many differential geometers studied different types of manifolds with a semi-symmetric metric connection. In the present paper, we study semi-symmetric nonmetric connections on an almost para-contact manifold in relation to semi-symmetric nonmetric connections. In another section of the work, we have studied the curvature tensor and Nijenhuis tensor.
Downloads
References
N. S. Agashe, M.R. Chaffle, “A semi-symmetric non-metric connection in a Riemannian manifold”, “Indian J.Pure and Applied Math.”, vol. 23(6), pp. 399-409, 1992.
T. Imai, “Notes on semi-symmetric metric connection”, “Tensor N.S.”, vol.24, pp. 293-296, 1972.
S.K. Srivastava, A.K. Srivastava, “On a semi-symmetric non metric connection in Lorentzian para sasakian manifold.”, “Journal of The Tensor Society”, Vol.-04, pp 103-112, 2010.
A. Sharfuddin, S.I. Hussain, “Semi-symmetric metric connections on almost contact manifold.”, “Tesnor N.S.”, vol. 30, pp. 133-139, 1976.
S. K. Srivastava, “Semi Symmtrician-metric connection on Manifold with generalized structure.”, “Journal of Ravishankar University”, Vol-15-16 pp. 49 to 58, 2002.
S.K. Srivastava, A. K. Srivastava, “Generalized quasi-Sasakian Manifold admitting Semi-symmetric Connection.”, “Journal of The Tensor Society”, Vol-2, pp. 83-89, 2008.
R. Nivas, J.P.Singh, “On submanifolds a Riemannian manifold with semi-symmetric non metric connection.”, “T.S.I.,India”, vol. 10,pp. 30-38, 1991.
A Burman, “On Lp Sasakian Manifolds admitting and Semi-symmetric non metric connection.”, “Kyungpook Mathematical journal”, Vol-58(1), pp. 105-116, 2018.
K.D. Singh, R. Singh, “On almost para contact metric manifolds with semi-symmetric metric connection.”, “Demostratic Math.”, vol VII No 2, pp. 331-341, 1979.
A. Burman, U.C. De, “Semi-symmetric non metric connection on Kenmotsu manifolds.”, “Romannian Journal of Mathematics and Computer Science”, Vol-5(1), pp. 13-24, 2015.
Downloads
Published
How to Cite
CITATION COUNT
Issue
Section
